Understanding And Making use of The S-Chart Management Limits Components: A Complete Information

Understanding and Making use of the s-Chart Management Limits Components: A Complete Information

Associated Articles: Understanding and Making use of the s-Chart Management Limits Components: A Complete Information

Introduction

With enthusiasm, let’s navigate by means of the intriguing matter associated to Understanding and Making use of the s-Chart Management Limits Components: A Complete Information. Let’s weave attention-grabbing data and supply contemporary views to the readers.

Understanding and Making use of the s-Chart Management Limits Components: A Complete Information

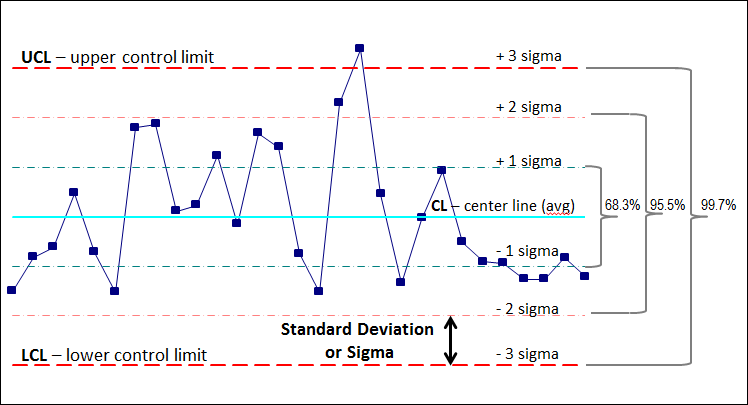
Statistical Course of Management (SPC) is an important software for monitoring and bettering the consistency of processes. Among the many varied management charts utilized in SPC, the s-chart performs an important function in monitoring the variability of a course of over time. Not like the x-bar chart which displays the method common, the s-chart focuses particularly on the usual deviation (or extra precisely, the pattern commonplace deviation, ‘s’) of subgroups of knowledge. Understanding the s-chart management limits system is paramount to successfully utilizing this highly effective software.

This text gives a complete exploration of the s-chart management limits system, its derivation, purposes, assumptions, and limitations. We’ll delve into the sensible facets of developing and deciphering s-charts, offering clear examples and highlighting the important concerns for profitable implementation.

Understanding the Idea of the s-Chart

The s-chart is a management chart used to watch the variability inside subgroups of knowledge. Every level plotted on the s-chart represents the pattern commonplace deviation (‘s’) calculated from a subgroup of knowledge collected at a selected time or location. The chart visually shows the variation within the course of over time, permitting for the identification of shifts in variability which may sign course of instability. Constant variability is a key indicator of a steady and predictable course of. Extreme or unpredictable variability typically factors in the direction of assignable causes that should be investigated and corrected.

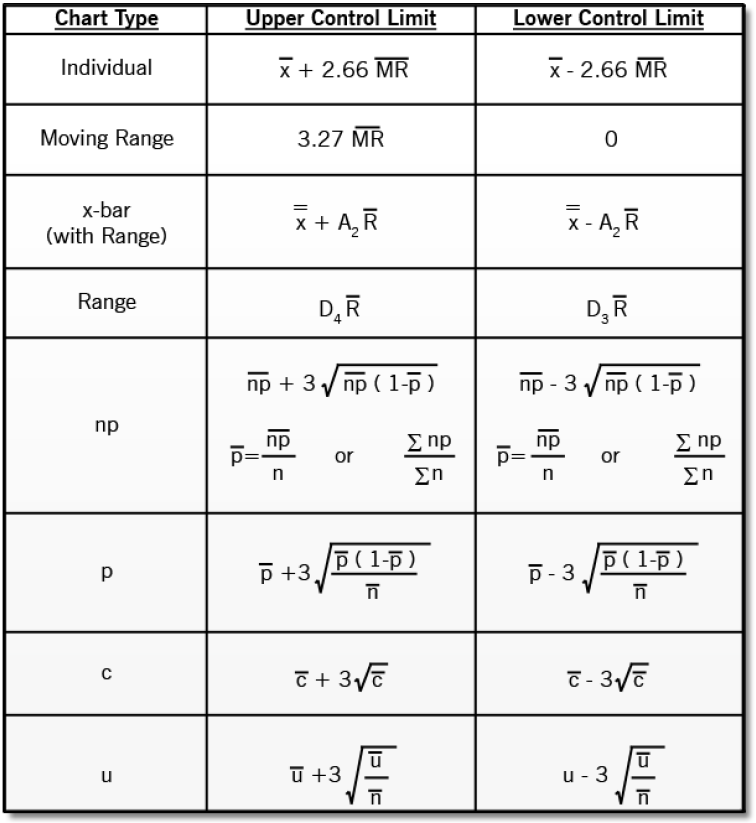
The Components for s-Chart Management Limits

The management limits for an s-chart are calculated utilizing the typical of the pattern commonplace deviations and an element derived from the chi-square distribution. The formulation are:

• *Higher Management Restrict (UCL): B₄ ¯s**
• Middle Line (CL): ¯s
• *Decrease Management Restrict (LCL): B₃ ¯s**

The place:

• ¯s (s-bar): The typical of the pattern commonplace deviations calculated from ‘okay’ subgroups. It is calculated as: ¯s = Σsᵢ / okay, the place sᵢ represents the usual deviation of the i-th subgroup.
• B₃ and B₄: Management chart constants that rely on the subgroup dimension (n). These constants are obtained from tables of management chart constants or calculated utilizing statistical software program. They’re based mostly on the chi-square distribution and account for the variability inherent in estimating the usual deviation from samples. B₃ is at all times lower than or equal to 1 and B₄ is at all times larger than or equal to 1. For small subgroup sizes (n<5), B₃ may be 0, leading to a LCL of 0.

Derivation of the Components

The derivation of the s-chart management limits is predicated on the chi-square distribution. The sum of squared deviations from the imply, divided by the inhabitants variance (σ²), follows a chi-square distribution with (n-1) levels of freedom. The pattern variance (s²) is an estimator of the inhabitants variance (σ²).

The management limits are derived by discovering the values of the chi-square distribution that correspond to a specified chance (usually 99.73%, or 3 commonplace deviations from the imply in a traditional distribution). These values are then used to find out the higher and decrease bounds for the pattern commonplace deviation (s). The constants B₃ and B₄ are derived from these chi-square values and are standardized to facilitate their use within the management restrict formulation.

Selecting the Subgroup Measurement (n)

The selection of subgroup dimension (n) is essential for the effectiveness of the s-chart. A bigger subgroup dimension gives a extra exact estimate of the usual deviation, however it additionally will increase the price and time required for information assortment. A smaller subgroup dimension is simpler to handle however is perhaps much less delicate to small shifts in variability. The optimum subgroup dimension relies on the particular software and the traits of the method being monitored. Typically, subgroup sizes between 4 and 10 are really useful.

Developing an s-Chart: A Step-by-Step Information

1. Accumulate Knowledge: Collect information in subgroups of dimension ‘n’ from the method. Be certain that the information is consultant of the method and picked up persistently over time.

2. Calculate Pattern Customary Deviations: Calculate the usual deviation (sᵢ) for every subgroup. Many statistical software program packages can automate this calculation.

3. Calculate the Common Customary Deviation (¯s): Sum the pattern commonplace deviations and divide by the variety of subgroups (okay).

4. Decide B₃ and B₄: Receive the values of B₃ and B₄ from a management chart constants desk based mostly on the subgroup dimension (n).

5. Calculate Management Limits: Use the formulation above to calculate the UCL, CL, and LCL.

6. Plot the Knowledge: Plot the pattern commonplace deviations (sᵢ) on a graph with the management limits.

7. Interpret the Chart: Analyze the chart to establish any factors outdoors the management limits or patterns that recommend course of instability.

Decoding the s-Chart

• Factors Exterior Management Limits: Factors outdoors the management limits point out that the method variability is unusually excessive or low. This warrants an investigation to establish and proper the assignable causes accountable for the variation.

• Tendencies and Patterns: Tendencies (consecutive factors growing or reducing) or patterns (e.g., cyclical variations) throughout the management limits may point out course of instability, even when no factors fall outdoors the boundaries. These patterns ought to be investigated to find out their root trigger.

• Secure Course of: If all factors fall throughout the management limits and there are not any apparent tendencies or patterns, it means that the method variability is steady and predictable.

Assumptions and Limitations of the s-Chart

The s-chart depends on a number of assumptions:

• Knowledge normality: The info inside every subgroup ought to be roughly usually distributed. Whereas the s-chart is comparatively sturdy to departures from normality, extreme deviations can have an effect on its accuracy.

• Independence of subgroups: The subgroups ought to be unbiased of one another. If subgroups are correlated, the management limits is not going to be correct.

• Fixed variability: The method variability ought to stay fixed over time. If the variability adjustments considerably, the management limits will change into invalid.

• Random sampling: The info ought to be collected by means of random sampling to make sure that it’s consultant of the method.

Options to the s-Chart

Whereas the s-chart is extensively used, there are different strategies for monitoring course of variability, together with:

• Vary Chart (R-chart): The R-chart makes use of the vary of every subgroup as a measure of variability. It is less complicated to calculate than the s-chart however much less environment friendly in detecting small shifts in variability.

• Transferring Vary Chart: This chart is especially helpful when subgroup sizes are small or when particular person observations can be found as a substitute of subgroups.

The selection of which chart to make use of relies on components such because the subgroup dimension, the information distribution, and the particular objectives of the management charting effort.

Conclusion

The s-chart is a priceless software for monitoring course of variability and making certain constant product high quality. Understanding the s-chart management limits system, its derivation, and its assumptions is essential for its efficient software. By rigorously gathering information, calculating the management limits appropriately, and deciphering the chart appropriately, practitioners can use the s-chart to establish and handle sources of variability, resulting in course of enhancements and enhanced product high quality. Do not forget that the s-chart is only when used at the side of different SPC instruments and a complete understanding of the method being monitored. Correct coaching and a methodical method are important for profitable implementation and interpretation of s-charts.

Closure

Thus, we hope this text has offered priceless insights into Understanding and Making use of the s-Chart Management Limits Components: A Complete Information. We admire your consideration to our article. See you in our subsequent article!